Painstaking Remake of History's Earliest Elevation Map

A British artist is tracing the curious invention of contour lines.
CityLab

LAURA BLISS
@mslaurabliss
Jun 9, 2016

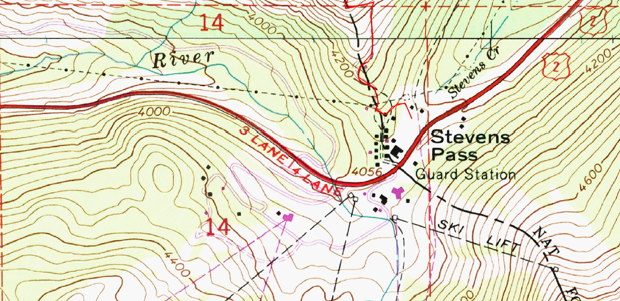An example of a modern-day relief map using contour lines. (Flickr/Bravenboer)

You’ve seen them on trail maps, road maps, and engineering plans. For centuries, contour lines have been a standard cartographic convention. Used to represent the elevation of land and mountains, to modern map-readers the curving marks are implicit representations of reality, as true as the blue of an oceanic map.

But as with most things in this human-built world, contour lines had to be invented. Their origins lie with Charles Hutton, a British mathematician whose ambitious 1774 survey of a Scottish peak called Schiehallion marked their first known use. That map was lost to history, but his original charts and tables of survey points were not.

Now, a British visual artist has painstakingly recreated Hutton’s map as part of her intensive online investigation of the history of the contour line. Karen Rannused Hutton’s centuries-old data to create a four-foot-square map of Schiehallion, mimicking Hutton’s draftsmanship and handwriting. She also turned those data points into an elegant 3-D model. Alongside artifacts from Hutton’s research, both of these cartographic works of art are on view at the Literary and Philosophical Society in Newcastle, where Rann currently lives and where Hutton once called home.

It’s an effort to resuscitate the importance of Hutton and his contribution to mathematics and cartography, says Rann, who learned about Hutton’s story from a short television program.

“I thought there would be a book about the invention of contour lines, but no one has written it,” she tells CityLab. “And the map that Charles Hutton had drawn is missing. There was this whole detective story and nobody was bothering with it.”

A detail from Rann’s re-created map. (Courtesy of Karen Rann)

It’s an enchanting story of the Enlightenment era. Hutton didn’t invent contour lines in order to represent Schiehallion’s 3,500-foot height. Rather, he created them to solve a problem with which he’d been tasked: weighing the mountain.

This project was all part of a grant from the Royal Society to calculate the weight of the Earth using Newton’s theory of gravitation, led by the astronomer Nevil Maskelyne. This required several challenging steps. The first involved dangling perfectly vertical pendulums from the mountain’s peak to measure the gravitational pull of the mountain, and determining from that, following Newton, its weight. The second was to calculate the volume and density of the mountain, which the scientists would then scale up to weigh the entire planet.

How would you set about weighing a mountain? Perhaps inspired by isobath lines—which had been already been used to show water depth—Hutton broke his task down into parts. The Institute of Mathematics and its Applications, a U.K. professional society, explains:


[Hutton] divided the mountain up into horizontal slices at regular intervals and worked out the volume of each slice. This was relatively straight forward as the mountain was chosen for its symmetrical nature. He then simply added the volume of each slice together to give the volume of the whole mountain. In [mapping this out], he had invented the idea of contour lines still used to this day.

Impressively, Maskelyne and Hutton did manage to estimate the weight of the planet within a 20 percent margin of error, and the Schiehallion experiment has gone down as one of the most influential in history. By the 19th century, Hutton’s contour lines would be adapted by European mapmakers to depict elevation—an essential referent for the industrial era’s new focus on mining and building railways and roads.

Rann’s 3D model of Schiehallion, according to Hutton’s contour lines. (Courtesy of Karen Rann)

But Hutton’s contributions have flown under the radar of history, says Rann, who can’t completely explain why she felt such a kinship to him. It’s partly their shared homeland, partly the fact that she just loves maps. “It’s hit a chord with a lot of people here, as well,” she says.

But on her blog, Rann waxes a little more poetic about what might make contour lines so enchanting:

Maps are usually drawn to define boundaries, often for defence or attack; or they are drawn for travellers, or landowners wanting to quantify what they possess. These maps are filled with symbols that represent things visible in that landscape: woods and windmills, roads, rivers, bridges and mountain ranges.

The maps explored here were the first to include those tentative lines that expose a quality of our world needing to be understood, to be calculated or defined, through the use of a line or lines which are not visible within the landscape because they are not there.

Hutton may not have intended to invent the contour line, but somewhat like Newton, he showed the world an invisible quality that was indispensable for understanding the world. Map-readers are forever grateful. 

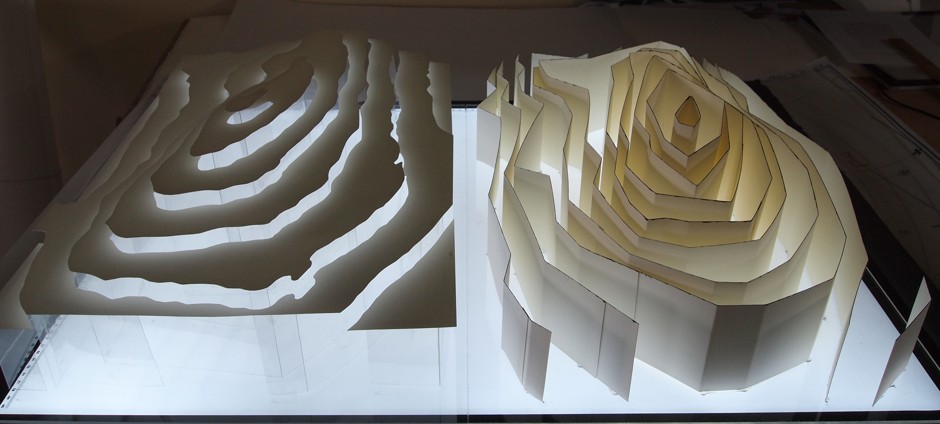Rann’s 3D model of Schiehallion, according to modern Ordnance Survey contour lines (left), compared to Rann’s 3D model of Schiehallion following Hutton’s contour lines. (Courtesy of Karen Rann)

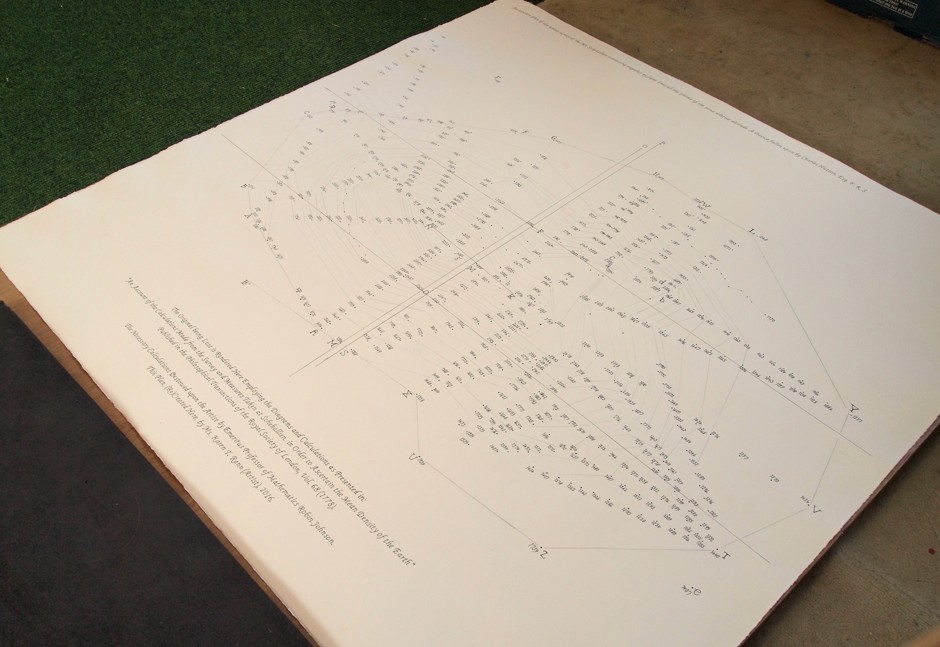Karen Rann’s re-creation of the original Hutton survey map. (Courtesy of Karen Rann)

Maps have "north" at the top but it could have been different

BBC
Caroline Williams

15 June 2016

Imagine looking at the Earth from space. What is at the top of the planet? If you said the North Pole, you probably wouldn’t be alone. Strictly speaking, you wouldn’t be right either.

The uncomfortable truth is that despite almost everybody imagining that the world is this way up, there is no good, scientific reason to think of north as being the roof of the world.

The story of how it came to be considered to be that way is heady mix of history, astrophysics and psychology. And it leads to an important conclusion: it turns out that the way we have decided to map the world has very real consequences for how we feel about it.

Navigating brain

Understanding where you are in the world is a basic survival skill, which is why we, like most species come hard-wired with specialised brain areas to create cognitive maps of our surroundings. Where humans are unique, though, with the possible exception of honeybees, is that we try to communicate this understanding of the world with others. We have a long history of doing this by drawing maps – the earliest versions yet discovered were scrawled on cave walls 14,000 years ago. Human cultures have been drawing them on stone tablets, papyrus, paper and now computer screens ever since.


"It is only within the last few hundred years that north has been consistently at the top"

Given such a long history of human map-making, it is perhaps surprising that it is only within the last few hundred years that north has been consistently considered to be at the top. In fact, for much of human history, north almost never appeared at the top, according to Jerry Brotton, a map historian from Queen Mary University, London and author of A History of the World in Twelve Maps. “North was rarely put at the top for the simple fact that north is where darkness comes from,” he says. “West is also very unlikely to be put at the top because west is where the sun disappears.”

Confusingly, early Chinese maps seem to buck this trend. But, Brotton, says, even though they did have compasses at the time, that isn’t the reason that they placed north at the top. Early Chinese compasses were actually oriented to point south, which was considered to be more desirable than deepest darkest north. But in Chinese maps, the Emperor, who lived in the north of the country was always put at the top of the map, with everyone else, his loyal subjects, looking up towards him. “In Chinese culture the Emperor looks south because it’s where the winds come from, it’s a good direction. North is not very good but you are in a position of subjection to the emperor, so you look up to him,” says Brotton.





The Kangnido map, a Chinese-influenced Korean map from 1402 (Credit: Wikipedia)


Given that each culture has a very different idea of who, or what, they should look up to it’s perhaps not surprising that there is very little consistency in which way early maps pointed. In ancient Egyptian times the top of the world was east, the position of sunrise. Early Islamic maps favoured south at the top because most of the early Muslim cultures were north of Mecca, so they imagined looking up (south) towards it:





Muhammad Al Idrissi’s map Tabula Rogeriana from 1154, upside down with north at the top (Credit: Wikipedia)


Christian maps from the same era (called Mappa Mundi) put east at the top, towards the Garden of Eden and with Jerusalem in the centre.





The Hereford Mappa Mundi from 1300 (Credit: Wikipedia)


So when did everyone get together and decide that north was the top? It’s tempting to put it down to European explorers like Christopher Columbus and Ferdinand Megellan, who were navigating by the North Star. But Brotton argues that these early explorers didn’t think of the world like that at all. “When Columbus describes the world it is in accordance with east being at the top,” he says. “Columbus says he is going towards paradise, so his mentality is from a medieval mappa mundi.” We’ve got to remember, adds Brotton, that at the time, “no one knows what they are doing and where they are going”.


"Mercator’s world map, from 1569, was a defining moment in north-up map-making"

Mercator’s world map, from 1569, was almost certainly a defining moment in north-up map-making. His map was famously the first to take into account the curvature of the Earth, so that sailors could cross long distances without overshooting the mark. Again, though, Brotton says that north had little to do with it. “Mercator projected the poles to infinity. He says in his description that it doesn’t matter because we are not terribly interested in sailing to them. North is at the top but nobody cares about north because we’re not going there.”

Even so, he could have put the map either way up. Perhaps the choice was simply because the Europeans were doing most of the exploring at the time: in the northern hemisphere, there is far more land to explore and far more people.





The 1569 Mercator map of the world (Credit: Wikipedia)


Whatever the reasons, north up is an idea that seems to have stuck. Take this famous Nasa image from 1973. This photograph was actually taken with south at the top, because the astronaut who took it was spinning around at the time. Nasa decided to flip it over to avoid confusing people.





This image of Earth was photographed this way round, but flipped before publication (Credit: Nasa)


When you start looking at the Earth from space though, the idea of it being any particular way up starts to make even less sense. It’s true that, as we all learned in school, the Earth lines up along the same plane as all of the other planets in the solar system because they all formed out of the same cloud of spinning dust. It is also true, though, that this picture could just as easily be put upside down or with the Sun at the top or bottom, depending on where in space you happen to be looking from.





(Credit: Nasa)

And compared to the rest of the Milky Way, our entire solar system is off kilter by about 63 degrees.

While astronomers have found that stars and planets align with their neighbours in similar ways all over space, Daniel Mortlock, an astrophysicist at Imperial College London, says that this is only true at a tiny scale compared to the vastness of the Universe. “As far as we astronomers can tell, there really is no ‘up’ or ‘down’ in space,” he says.

So the answer to the question of which way up is the Earth is simple: it is not any particular way up and there is no good reason other than a historical superiority complex to think of north as being the top of the world.

Yet is it time to start embracing a different view of the planet from the one we are used to? Perhaps, because evidence from psychology suggests that our north-up culture might be polluting the way we think of what is valuable in the world.





Could there be a northern bias that shapes how we think about some parts of the planet? (Credit: Nasa)


A well-known bias in psychology reveals that most people think of north as being ‘up’ and south, ‘down’. Brian Meier, a psychologist at Gettysberg College in Pennsylvania, has also found that people unconsciously process positive words as if they were higher in space than negative ones. So he wondered whether these two things, north = up and good = up affect the value that people put on different areas on a map.

It isn’t too much of a stretch to think that people are less likely to care about countries that are ‘lower’ than them

Sure enough, when shown a map of a hypothetical city and asked where they would like to live, people were significantly more likely to choose an area in the north of the city. And when another group of people were asked where fictitious people of different social status would live, they plotted them on the map with the richest in the north and poorest in the south.

It isn’t too much of a stretch to think that people are less likely to care what happens in countries or regions that are ‘lower’ than them on the map or globe.

The good news is that in Meier’s experiments the relationship between ‘north’ and ‘good’ was eliminated by one simple thing – turning the map upside down. So perhaps the world might get a little fairer if we just took a look at it another way up now and again. South-up maps are easily available online. It is also something that Mortlock is very much in favour of: “As an Australian, I think it should be done more often,” he says.

If nothing else, it’s a sure-fire way to make the world seem fresh, and unexplored, once more. With so few earthbound discoveries left for our generation to make, all we can do is – to paraphrase Marcel Proust – look again at the world we’ve got, but this time, through different eyes.